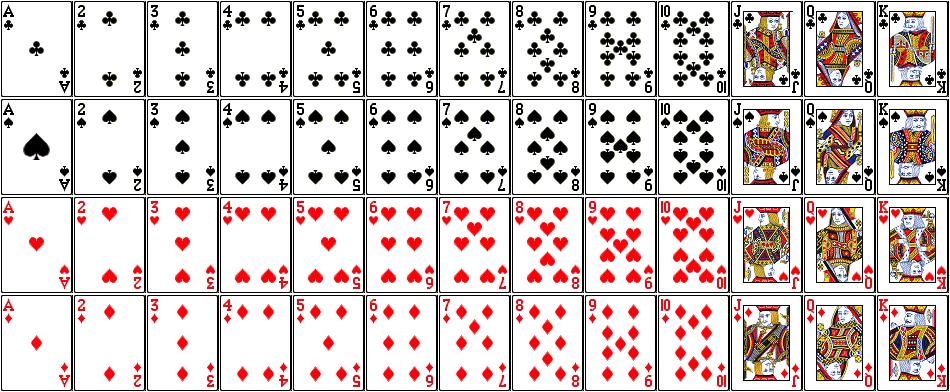
In a pack or deck of 52 playing cards, they are divided into 4 suits of 13 cards each i.e. spades ♠ hearts ♥, diamonds ♦, clubs ♣.
Total Blck Cards = 26
Total Red Cards = 26
Total Ace Cards = 4
Total Face cards = 12
Total Spade Cards = 13
Total Club cards = 13
Total Diamond Cards = 13
Total Hearts cards = 13
Total Jack Cards = 4
Total Queen Cards = 4
Total King Cards = 4
Face cards: Jacks, Queens, and Kings are called "face cards" because the cards have pictures of their names.
Question: A card is drawn from a well shuffled pack of 52 cards. Find the probability of:
(i) ‘2’ of spades.
(ii) A jack.
(iii) A king of red colour.
(iv) A card of diamond.
(v) A king or a queen.
(vi) A non-face card.
(vii) A black face card.
(viii) A black card.
(ix) A non-ace.
(x) Non-face card of black colour.
(xi) Neither a spade nor a jack.
(xii) Neither a heart nor a red king.
Solution:
In a playing card there are 52 cards.
Therefore the total number of possible outcomes = 52
(i) ‘2’ of spades:
Number of favourable outcomes i.e. ‘2’ of spades is 1 out of 52 cards.
Therefore, probability of getting ‘2’ of spade
Number of favorable outcomes
P(A) = Total number of possible outcome
= 1/52
(ii) A jack:
Number of favourable outcomes i.e. ‘a jack’ is 4 out of 52 cards.
Therefore, probability of getting ‘a jack’
Number of favorable outcomes
P(B) = Total number of possible outcome
= 4/52
= 1/13
(iii) A king of red colour:
Number of favourable outcomes i.e. ‘a king of red colour’ is 2 out of 52 cards.
Therefore, probability of getting ‘a king of red colour’
Number of favorable outcomes
P(C) = Total number of possible outcome
= 2/52
= 1/26
(iv) A card of diamond:
Number of favourable outcomes i.e. ‘a card of diamond’ is 13 out of 52 cards.
Therefore, probability of getting ‘a card of diamond’
Number of favorable outcomes
P(D) = Total number of possible outcome
= 13/52
= 1/4
(v) A king or a queen:
Total number of king is 4 out of 52 cards.
Total number of queen is 4 out of 52 cards
Number of favourable outcomes i.e. ‘a king or a queen’ is 4 + 4 = 8 out of 52 cards.
Therefore, probability of getting ‘a king or a queen’
Number of favorable outcomes
P(E) = Total number of possible outcome
= 8/52
= 2/13
(vi) A non-face card:
Total number of face card out of 52 cards = 3 times 4 = 12
Total number of non-face card out of 52 cards = 52 - 12 = 40
Therefore, probability of getting ‘a non-face card’
Number of favorable outcomes
P(F) = Total number of possible outcome
= 40/52
= 10/13
(vii) A black face card:
Cards of Spades and Clubs are black cards.
Number of face card in spades (king, queen and jack or knaves) = 3
Number of face card in clubs (king, queen and jack or knaves) = 3
Therefore, total number of black face card out of 52 cards = 3 + 3 = 6
Therefore, probability of getting ‘a black face card’
Number of favorable outcomes
P(G) = Total number of possible outcome
= 6/52
= 3/26
(viii) A black card:
Cards of spades and clubs are black cards.
Number of spades = 13
Number of clubs = 13
Therefore, total number of black card out of 52 cards = 13 + 13 = 26
Therefore, probability of getting ‘a black card’
Number of favorable outcomes
P(H) = Total number of possible outcome
= 26/52
= 1/2
(ix) A non-ace:
Number of ace cards in each of four suits namely spades, hearts, diamonds and clubs = 1
Therefore, total number of ace cards out of 52 cards = 4
Thus, total number of non-ace cards out of 52 cards = 52 - 4
= 48
Therefore, probability of getting ‘a non-ace’,
Number of favorable outcomes
P(I) = Total number of possible outcome
= 48/52
= 12/13
(x) Non-face card of black colour:
Cards of spades and clubs are black cards.
Number of spades = 13
Number of clubs = 13
Therefore, total number of black card out of 52 cards = 13 + 13 = 26
Number of face cards in each suits namely spades and clubs = 3 + 3 = 6
Therefore, total number of non-face card of black colour out of 52 cards = 26 - 6 = 20
Therefore, probability of getting ‘non-face card of black colour’,
Number of favorable outcomes
P(J) = Total number of possible outcome
= 20/52
= 5/13
(xi) Neither a spade nor a jack:
Number of spades = 13
Total number of non-spades out of 52 cards = 52 - 13 = 39
Number of jack out of 52 cards = 4
Number of jack in each of three suits namely hearts, diamonds and clubs = 3
[Since, 1 jack is already included in the 13 spades so, here we will take number of jacks is 3]
Neither a spade nor a jack = 39 - 3 = 36
Therefore, probability of getting ‘neither a spade nor a jack’,
Number of favorable outcomes
P(K) = Total number of possible outcome
= 36/52
= 9/13
(xii) Neither a heart nor a red king:
Number of hearts = 13
Total number of non-hearts out of 52 cards = 52 - 13 = 39
Therefore, spades, clubs and diamonds are the 39 cards.
Cards of hearts and diamonds are red cards.
Number of red kings in red cards = 2
Therefore, neither a heart nor a red king = 39 - 1 = 38
[Since, 1 red king is already included in the 13 hearts so, here we will take number of red kings is 1]
Therefore, probability of getting ‘neither a heart nor a red king’
Number of favorable outcomes
P(L) = Total number of possible outcome
= 38/52
= 19/26
These are the basic problems on probability with playing cards.


 0
0

